Symmetry Lines II

When a polygon is flipped over a line of symmetry the resulting polygon is identical in shape and orientation to the original polygon. In the grid below, each column represents the number of lines of symmetry. Try to draw polygons that fit in each space on this grid. Many cannot be done! Place an X in the box if you think the specific case is not possible.

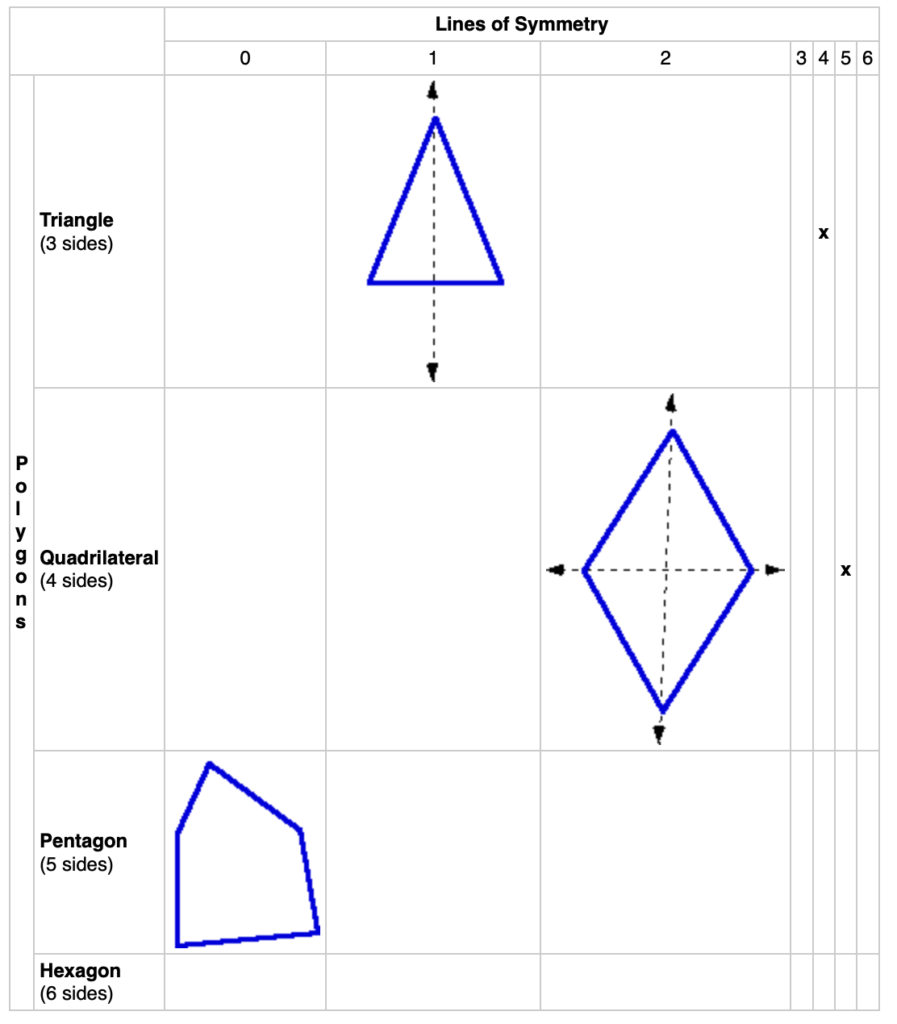
For a given number of sides, can you always make a polygon with no lines of symmetry? With one? Why?

Do you see any patterns that could help you predict which spaces can be filled in for seven-sided polygons?

What can you say about the lines of symmetry of regular polygons?

(Adapted with permission from Geometry in the Middle Grades, Addenda Series, copyright 1992 by the National Council of Teachers of Mathematics. All rights reserved.)